Cable and memedit]
Membrane materials[edit]
Common materials for doubly curved fabric structures are
PTFE-coated
fiberglass and
PVC-coated
polyester. These are woven materials with different strengths in different directions. The
warp fibers (those fibers which are originally straight—equivalent to the starting fibers on a loom) can carry greater load than the
weft or fill fibers, which are woven between the warp fibers.
Other structures make use of
ETFE film, either as single layer or in cushion form (which can be inflated, to provide good insulation properties or for aesthetic effect—as on the
Allianz Arena in
Munich). ETFE cushions can also be etched with patterns in order to let different levels of light through when inflated to different levels. They are most often supported by a structural frame as they cannot derive their strength from double curvature.

Simple suspended bridge working entirely in tension
Cables can be of
mild steel,
high strength steel (drawn carbon steel),
stainless steel,
polyester or
aramid fibres. Structural cables are made of a series of small strands twisted or bound together to form a much larger cable. Steel cables are either spiral strand, where circular rods are twisted together and "glued" using a polymer, or locked coil strand, where individual interlocking steel strands form the cable (often with a spiral strand core).
Spiral strand is slightly weaker than locked coil strand. Steel spiral strand cables have a
Young's modulus,
E of 150±10 kN/mm² (or 150±10
GPa) and come in sizes from 3 to 90 mm diameter. Spiral strand suffers from construction stretch, where the strands compact when the cable is loaded. This is normally removed by pre-stretching the cable and cycling the load up and down to 45% of the ultimate tensile load.
Locked coil strand typically has a Young's Modulus of 160±10 kN/mm² and comes in sizes from 20 mm to 160 mm diameter.
The properties of the individuals strands of different materials are shown in the table below, where UTS is
ultimate tensile strength, or the breaking load:
| E (GPa) | UTS (MPa) | Strain at 50% of UTS |
|---|
| Solid steel bar | 210 | 400–800 | 0.24% |
| Steel strand | 170 | 1550–1770 | 1% |
| Wire rope | 112 | 1550–1770 | 1.5% |
| Polyester fibre | 7.5 | 910 | 6% |
| Aramid fibre | 112 | 2800 | 2.5% |
Structural forms[edit]
Air-supported structures are a form of tensile structures where the fabric envelope is supported by pressurised air only.
The majority of fabric structures derive their strength from their doubly curved shape. By forcing the fabric to take on double-curvature
[1] the fabric gains sufficient
stiffness to withstand the loads it is subjected to (for example
wind and
snow loads). In order to induce an adequately doubly curved form it is most often necessary to pretension or prestress the fabric or its supporting structure.
Form-finding[edit]
The behaviour of structures which depend upon prestress to attain their strength is non-linear, so anything other than a very simple cable has, until the 1990s, been very difficult to design. The most common way to design doubly curved fabric structures was to construct scale models of the final buildings in order to understand their behaviour and to conduct
form-finding exercises. Such scale models often employed stocking material or tights, or soap film, as they behave in a very similar way to structural fabrics (they cannot carry shear).
Soap films have uniform stress in every direction and require a closed boundary to form. They naturally form a minimal surface—the form with minimal area and embodying minimal energy. They are however very difficult to measure. For large films the self-weight of the film can seriously and adversely affect the form.
For a membrane with curvature in two directions, the basic equation of equilibrium is:
where:
- R1 and R2 are the principal radii of curvature for soap films or the directions of the warp and weft for fabrics
- t1 and t2 are the tensions in the relevant directions
- w is the load per square metre
Lines of
principal curvature have no twist and intersect other lines of principal curvature at right angles.
A
geodesic or
geodetic line is usually the shortest line between two points on the surface. These lines are typically used when defining the cutting pattern seam-lines. This is due to their relative straightness after the planar cloths have been generated, resulting in lower cloth wastage and closer alignment with the fabric weave.
In a pre-stressed but unloaded surface
w = 0, so

.
In a soap film surface tensions are uniform in both directions, so R1 = −R2.
The final shape, or form, of a fabric structure depends upon:
- shape, or pattern, of the fabric
- the geometry of the supporting structure (such as masts, cables, ringbeams etc.)
- the pretension applied to the fabric or its supporting structure
It is important that the final form will not allow
ponding of water, as this can deform the membrane and lead to local failure or progressive failure of the entire structure.
Snow loading can be a serious problem for membrane structure, as the snow often will not flow off the structure as water will. For example, this has in the past caused the (temporary) collapse of the
Hubert H. Humphrey Metrodome, an air-inflated structure in
Minneapolis, Minnesota. Some structures prone to
ponding use heating to melt snow which settles on them.
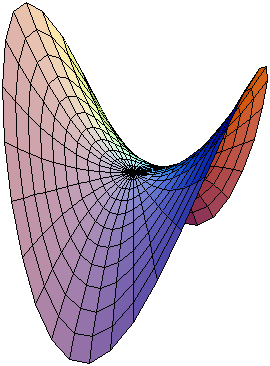
There are many different doubly curved forms, many of which have special mathematical properties. The most basic doubly curved from is the saddle shape, which can be a
hyperbolic paraboloid (not all saddle shapes are hyperbolic paraboloids). This is a double
ruled surface and is often used in both in lightweight shell structures (see
hyperboloid structures). True ruled surfaces are rarely found in tensile structures. Other forms are
anticlastic saddles, various radial, conical tent forms and any combination of them.
Pretension[edit]
Pretension is tension artificially induced in the structural elements in addition to any self-weight or imposed loads they may carry. It is used to ensure that the normally very flexible structural elements remain stiff under all possible loads.
A day to day example of pretension is a shelving unit supported by wires running from floor to ceiling. The wires hold the shelves in place because they are tensioned - if the wires were slack the system would not work.
Pretension can be applied to a membrane by stretching it from its edges or by pretensioning cables which support it and hence changing its shape. The level of pretension applied determines the shape of a membrane structure.
Alternative form-finding approach[edit]
The alternative approximated approach to the form-finding problem solution is based on the total energy balance of a grid-nodal system. Due to its physical meaning this approach is called the
Stretched Grid Method (SGM).
Simple mathematics of cables[edit]
Transversely and uniformly loaded cable[edit]
A uniformly loaded cable spanning between two supports forms a curve intermediate between a
catenary curve and a
parabola. The simplifying assumption can be made that it approximates a circular arc (of radius
R).
The horizontal and vertical reactions :


The length of the cable:

The tension in the cable:

By substitution:

The tension is also equal to:

The extension of the cable upon being loaded is (from
Hooke's Law, where the axial stiffness,
k, is equal to

):

If an initial pretension,

is added to the cable, the extension becomes:

Combining the above equations gives:

By plotting the left hand side of this equation against
T, and plotting the right hand side on the same axes, also against
T, the intersection will give the actual equilibrium tension in the cable for a given loading
w and a given pretension

.
Cable with central point load[edit]
A similar solution to that above can be derived where:
By equilibrium:


By geometry:

This gives the following relationship:

As before, plotting the left hand side and right hand side of the equation against the tension,
T, will give the equilibrium tension for a given pretension,

and load,
W.
Tensioned cable oscillations[edit]

where:
T = tension in
newtons,
m =
mass in kilograms and
L = span length.
Notable structures[edit]
- Shukhov Rotunda, Russia, 1896
- Canada Place, Vancouver, British Columbia for Expo '86
- Yoyogi National Gymnasium by Kenzo Tange, Yoyogi Park, Tokyo, Japan
- Ingalls Rink, Yale University by Eero Saarinen
- Khan Shatyry Entertainment Center, Astana, Kazakhstan
- Tropicana Field, St. Petersburg, Florida
- Olympiapark, Munich by Frei Otto
- Sidney Myer Music Bowl, Melbourne
- The O2 (formerly the Millennium Dome), London by Buro Happold and Richard Rogers Partnership
- Denver International Airport, Denver
- Dorton Arena, Raleigh
- Georgia Dome, Atlanta, Georgia by Heery and Weidlinger Associates
- Grantley Adams International Airport, Christ Church, Barbados
- Pengrowth Saddledome, Calgary by Graham McCourt Architects and Jan Bobrowski and Partners
- Scandinavium, Gothenburg, Sweden
- Hong Kong Museum of Coastal Defence
- Ashford Retail Village, Kent, UK, by Buro Happold, Richard Rogers and Architen Landrell
- Barclays Bank Headquarters, London
- Beckham Academy, London by Buro Happold
- Butlins Skyline Pavilion, Minehead, UK
- Carlos Moseley Music Pavilion, New York, NY
- Modernization of the Central Railway Station, Sofia, Bulgaria
- Columbus Center, Baltimore, Maryland
- Finnish Chancery, Washington, DC
- Imagination Headquarters, London
- National Symphony Orchestra, Washington, DC
- Pier6 Music Pavilion, Baltimore, Maryland
- Plashet Bridge, London by Birds Portchmouth Russum Architects
- Redbird Arena, Illinois State University, Normal, Illinois
- Desert Night resort, Wahiba Sands,Sultanate of Oman
- Retractable Umbrellas, Al-Masjid an-Nabawi, Medina, Saudi Arabia